Our research field is the physics of elementary particles and nuclei, which tries to answer questions as old as the history of mankind: What is the smallest component of matter, and what is matter’s most fundamental interaction? This research subject is related to the early universe and nucleosynthesis resulting from the Big Bang. Another important focus of ours is quantum properties, which play an essential role in the world of elementary particles and nuclei, as well as in material physics at the atomic or molecular level. We investigate nonperturbative properties of elementary particles and nuclei through numerical simulations with the use of lattice QCD (Quantum ChromoDynamics).
Successful numerical simulations heavily depend on raising computer performance by improving algorithms and computational techniques. However, that is a difficult challenge given the trend of computer architecture is to employ increasingly large-scale hierarchical parallel structures consisting of tens of thousands of nodes, which individually have increasing numbers of CPU cores and arithmetic accelerators with even higher degrees of parallelism. We need to develop new types of algorithms and computational techniques different from conventional types, to achieve better computer performance. We will overcome this difficult situation by collaborating with applied mathematicians and computer scientists.
Recent Achievements
Analyzing the phase structure of two-dimensional QED
The Tensor Network (TN) scheme has been evolving conceptually and practically over the past fifteen years, and has produced theoretical and computational tools in various fields. In elementary particle physics, we recently succeeded in applying the Grassmann Tensor Renormalization Group (GTRG) algorithm, a TN scheme, to the analysis of the phase structure of one-flavor lattice Schwinger model (two-dimensional QED). The results show that the algorithm is free from the sign problem, and the computational cost is comparable to bosonic case, thanks to the direct manipulation of the Grassmann variables. This was the first successful application of a TN scheme to a Euclidean lattice gauge-theory including relativistic fermions.
Furthermore, we also performed analysis on the phase structure of the one-flavor lattice Schwinger model with the so-called θ-term. In spite of the complex action, we have succeeded in reproducing the phase structure predicted by analytical calculations. This provides evidence that the GTRG algorithm has the ability to treat complex actions. Our work has shown that the GTRG algorithm solves the notorious sign problem inherent in the Monte Carlo method by using the Schwinger model as a testing ground. Toward the final goal of investigating four-dimensional lattice QCD, which is the SU(3) non-Abelian gauge theory with relativistic fermions, we are currently studying higher dimensional models including non-Abelian gauge theories.
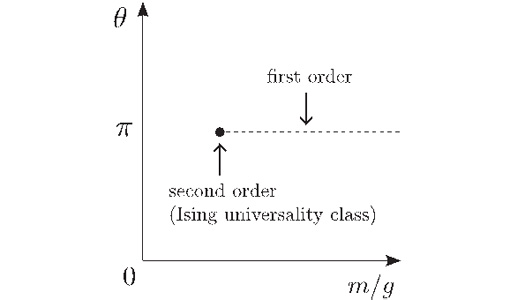
Phase diagram of one-flavor Schwinger model withθ-term




